Basic Shapes to Advanced Geometry
Apply increasingly complex rules of geometry to help an imprisoned mummy swing across pits and escape its pyramid prison.
Apply increasingly complex rules of geometry to help an imprisoned mummy swing across pits and escape its pyramid prison.
Curriculum-aligned activities
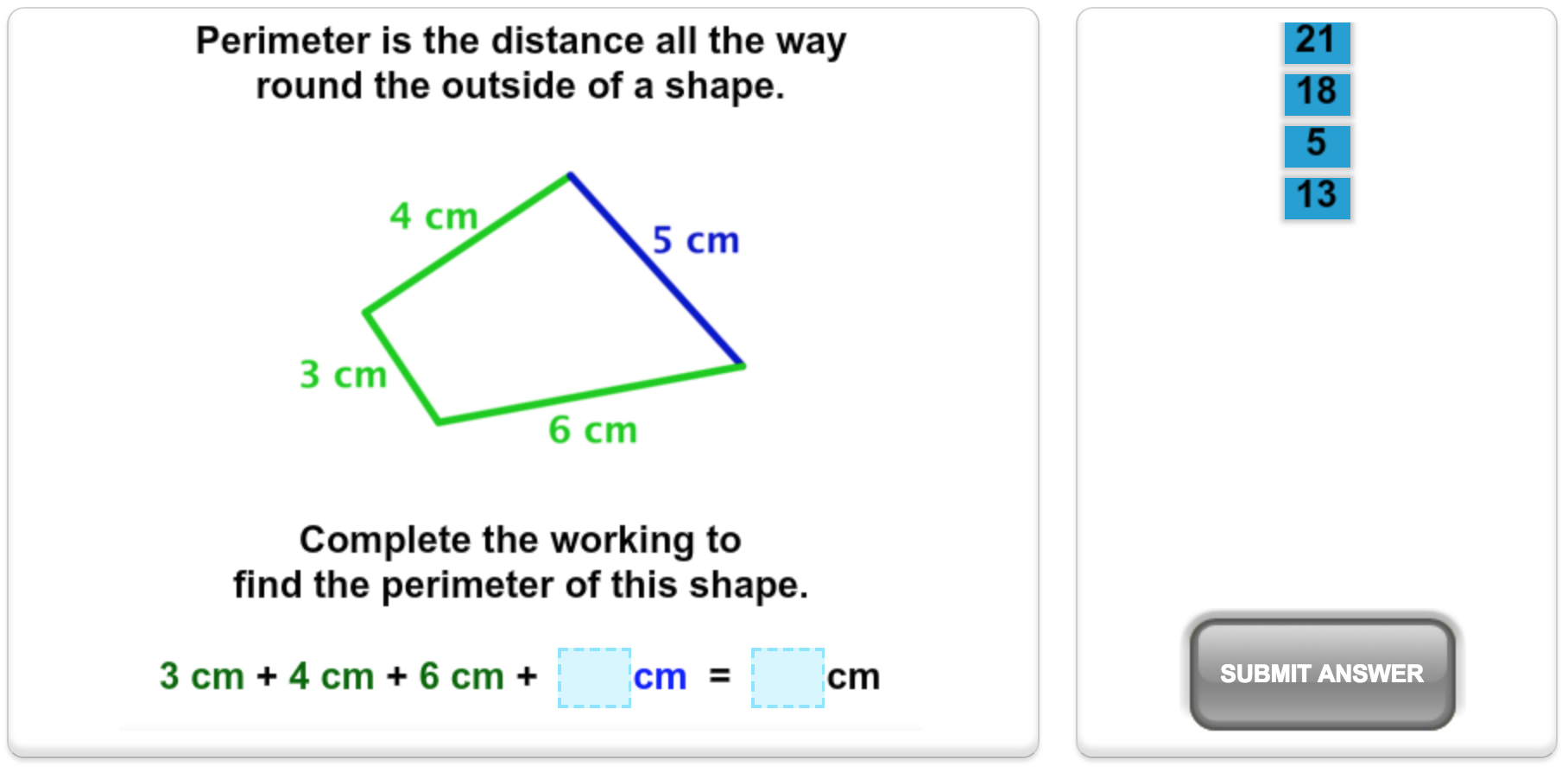
Understand that the perimeter means the total distance around a shape. Calculate the perimeter of polygons based on rectangles and triangles.
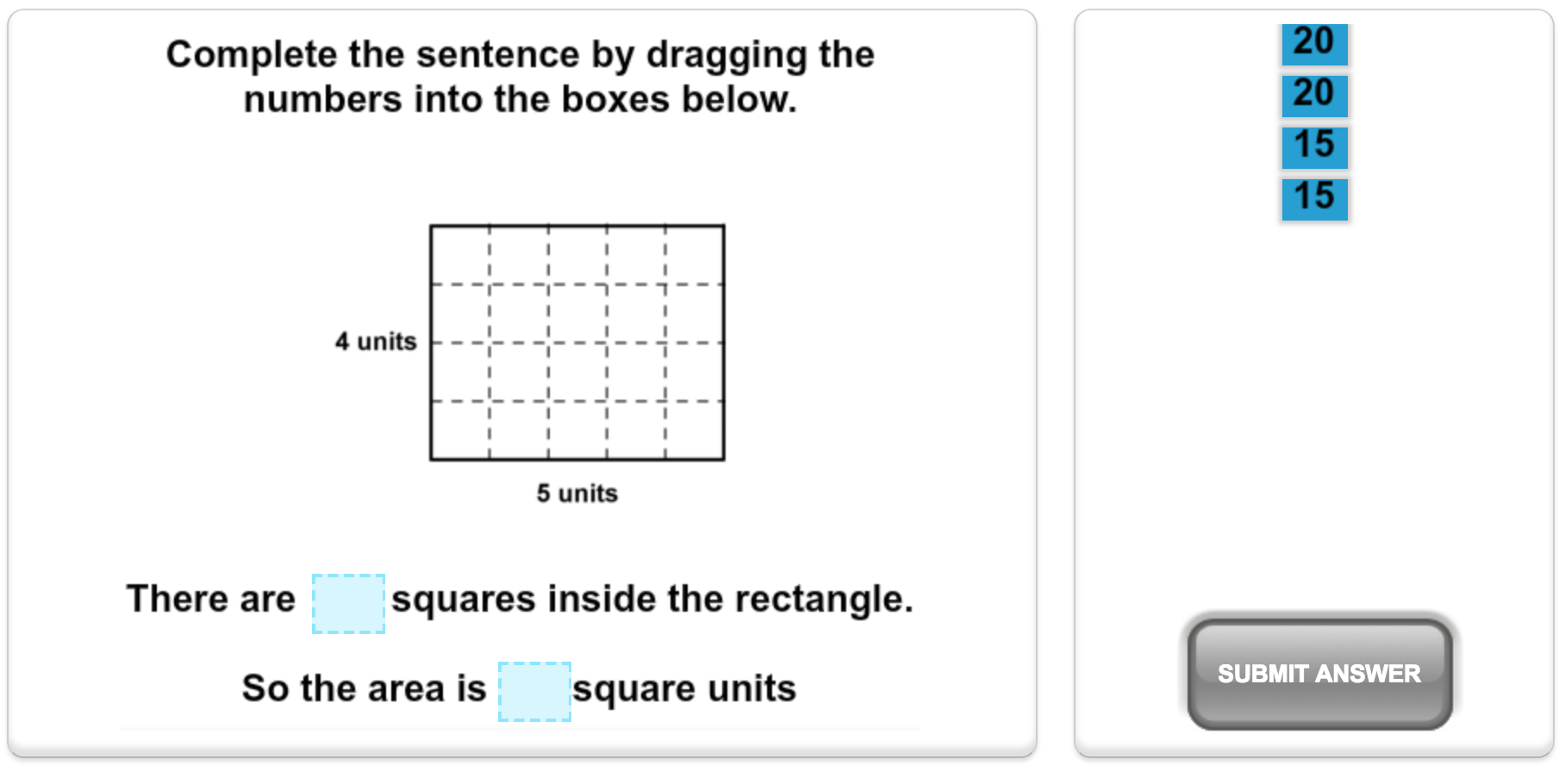
Use the formula for the area of a rectangle to calculate the rectangle's area.
Calculate the area of a triangle using the base length multiplied by the perpendicular height, then divided by 2. Include reverse problems e.g. find the perimeter or some side given the area. Derive the formula by considering the rectangle being halved.
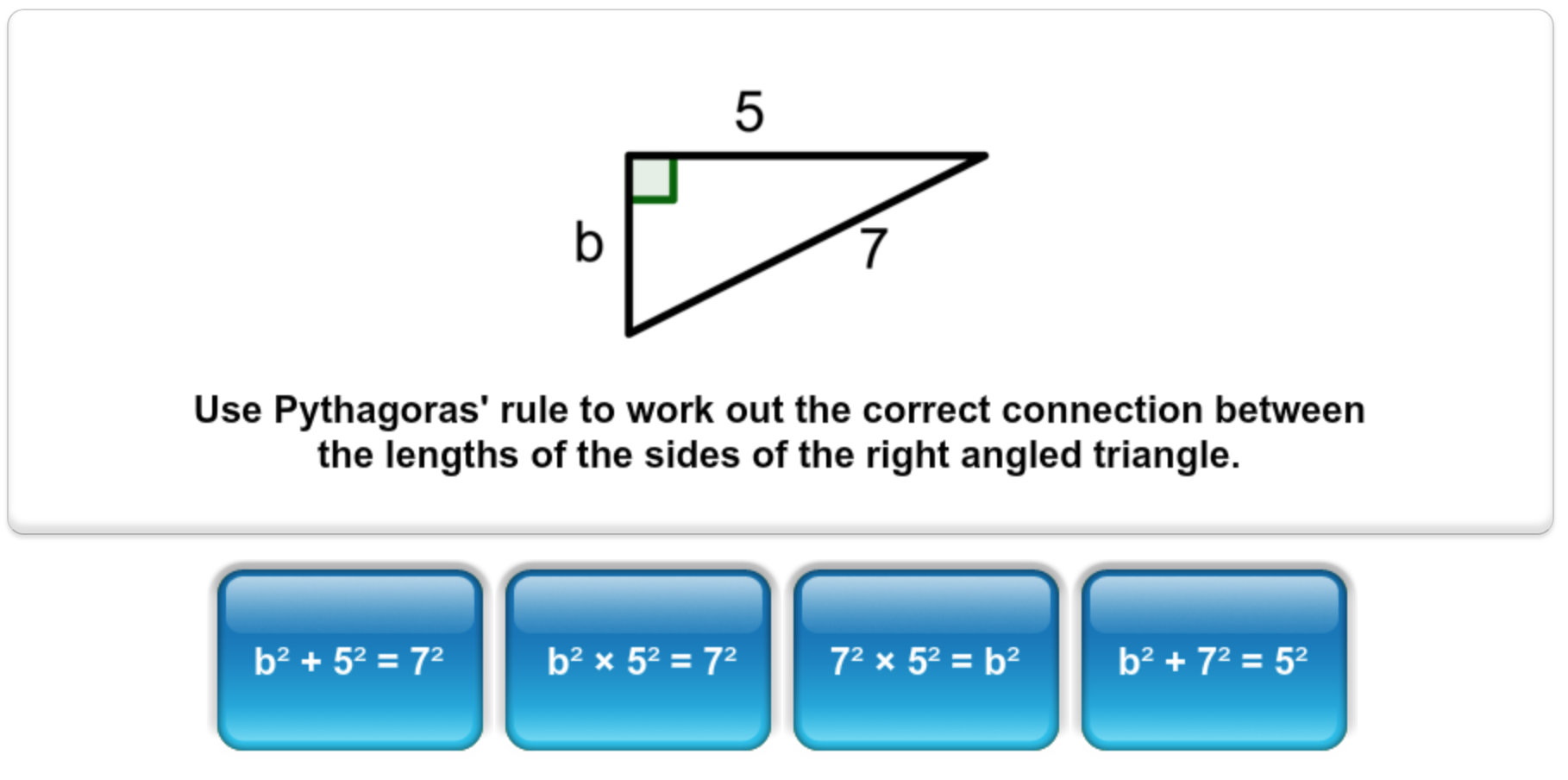
Know the formula for Pythagoras and use it to find the length of the longest side in a right angled triangle.
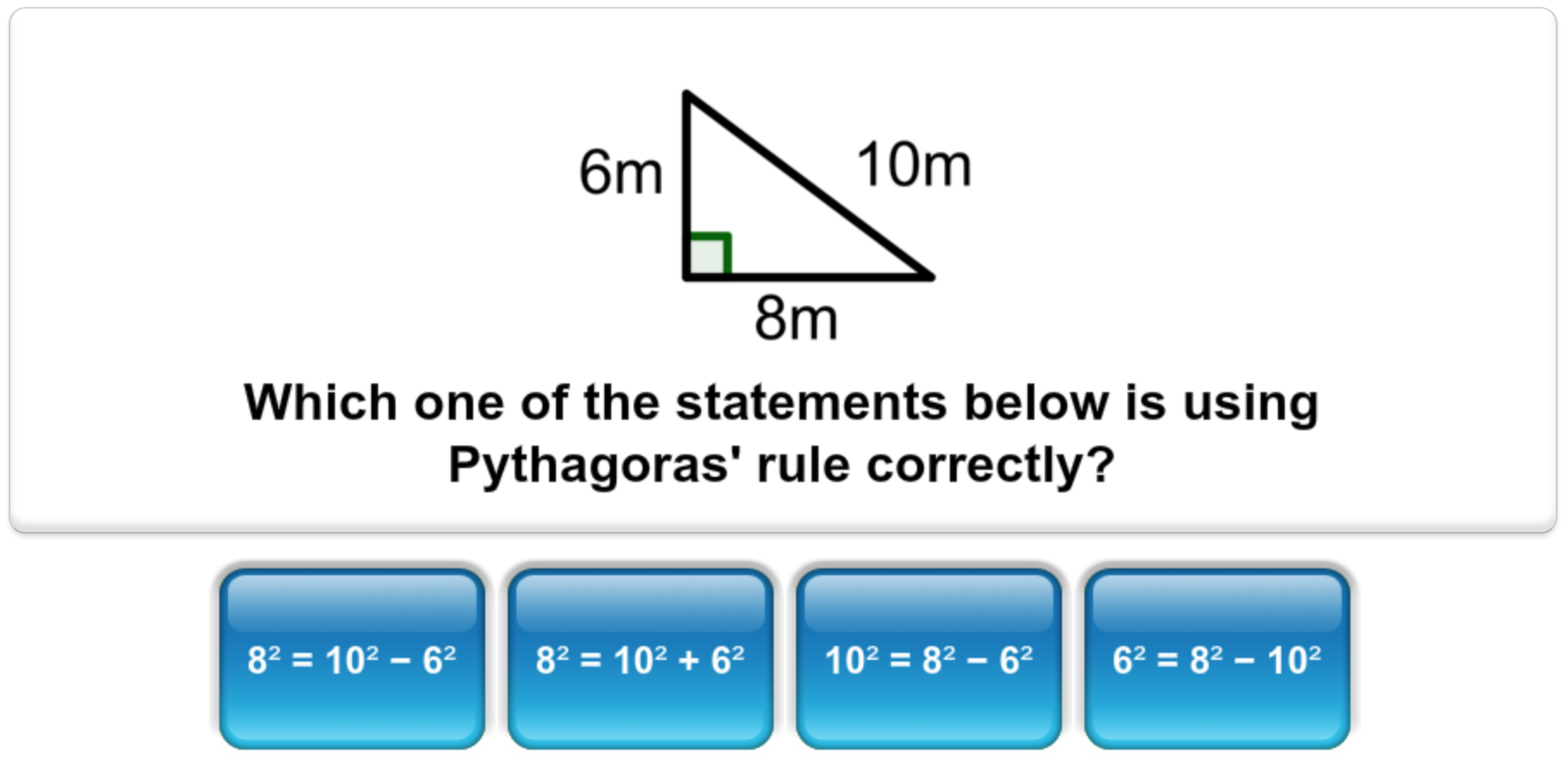
Know the formula for Pythagoras and use it to find the length of one of the shorter sides in a right angled triangle.
Use Pythagoras' theorem to find out if a triangle is right angled.
We’re loved by both teachers and students globally. Here’s the proof!
Mangahigh turns our students into “math addicts“ who compete with each other for top scores and gold medals. And since the quizzes reward both accurate recall of knowledge and deep conceptual understanding, every hour they spent having fun makes them better mathematicians. Five stars.
Ark Academy, Wembley, London
I have used Mangahigh in my classroom for over 5 years. What keeps me coming back are the math games and wide range of concepts that are offered. But the best part is the fact that the kids LOVE to play it. I have students beg me to assign them Teacher Challenges! Begging for more math work? I am ok with that!!
Green Elementary School, Allen, Texas
Kids loved it; an ADHD student who has NEVER before been able to focus in the last periods of the day; he wouldn't stop till he got a medal! Absolutely phenomenal! His mother is overjoyed, and the rest of the math staff room were gobsmacked!
Emanuel School, NSW
